Foci of an Ellipse

 

Foci of an Ellipse formula
\( c^2 \;=\; a^2 - b^2  \)
Symbol English Metric
\( c \) = length center to focus \( in \) \( mm \)
\( a \) = length semi-major axis \( in \) \( mm \)
\( b \) = length semi-minor axis \( in \) \( mm \)

ellipse foci 1

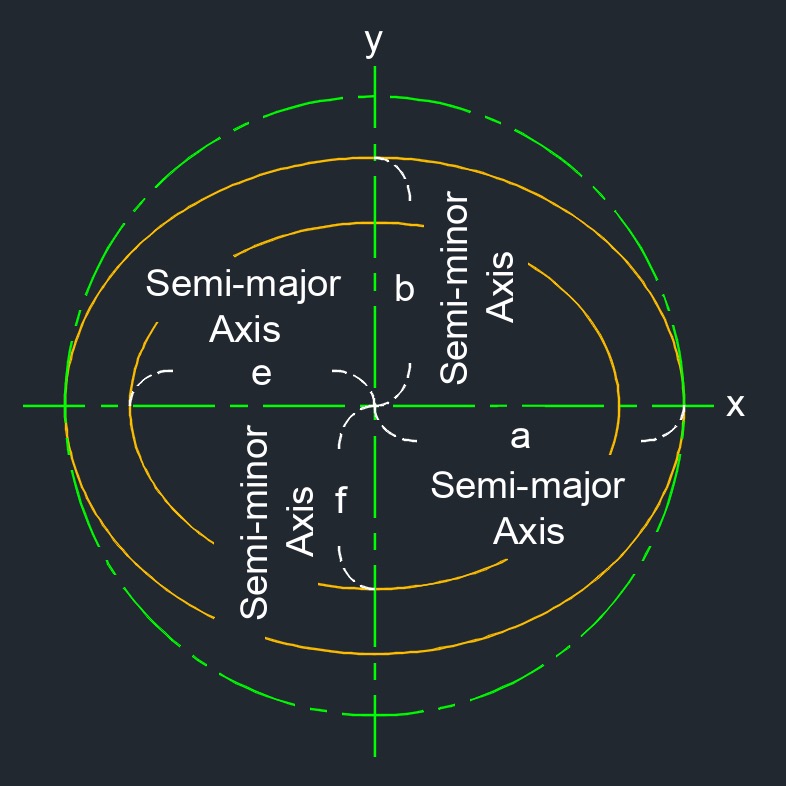
hollow ellipse s major s minor 3

 

 

 

 

 

  • Foci is a point used to define the conic section.  F and G seperately are called "focus", both togeather are called "foci". 

Piping Designer Logo 1