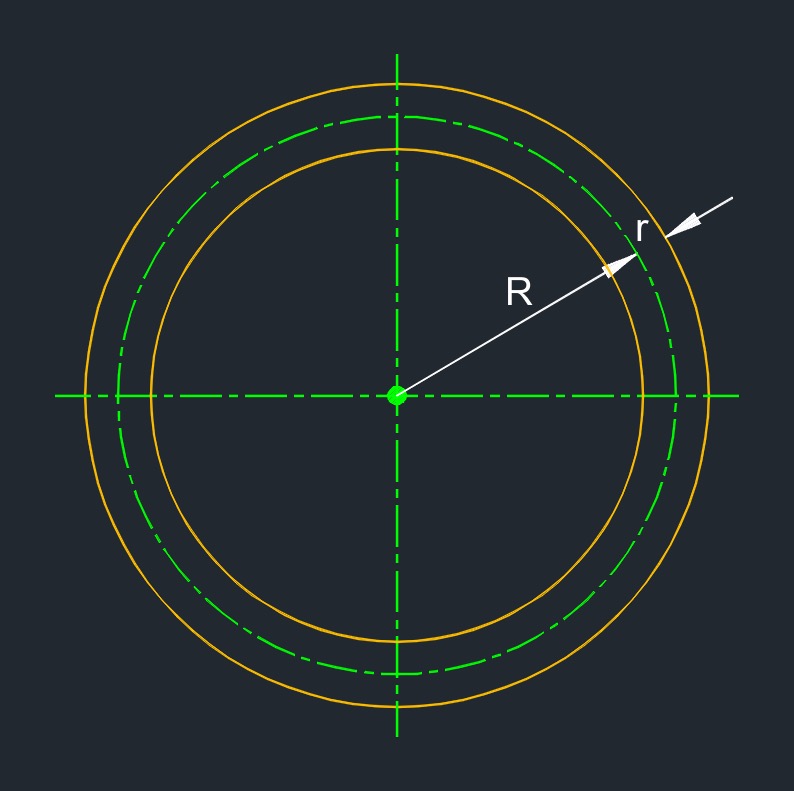
Hole Radius of a torus formula |
||
| \( R_h \;=\; R - r \) | ||
| Symbol | English | Metric |
| \( R_h \) = radius of the hole | \(in\) | \( mm \) |
| \( r \) = radius of sphere | \(in\) | \( mm \) |
| \( R \) = radius of center of sphere | \(in\) | \( mm \) |

Surface Area of a torus formula |
||
| \( S \;=\; 4 \cdot \pi^2 \cdot R \cdot r \) | ||
| Symbol | English | Metric |
| \( S \) = surface area | \( in^2 \) | \( mm^2 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | \(3.141 592 653 ...\) |
| \( r \) = radius of sphere | \(in\) | \( mm \) |
| \( R \) = radius of center of sphere | \(in\) | \( mm \) |
Volume of a torus formula |
||
| \( V \;=\; 2 \cdot \pi^2 \cdot R \cdot r^2 \) | ||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | \(3.141 592 653 ...\) |
| \( r \) = radius of sphere | \(in\) | \( mm \) |
| \( R \) = radius of center of sphere | \(in\) | \( mm \) |