Height of a Oblique Cylinder formula |
||
| \( h \;=\; l \cdot sin(x) \) | ||
| Symbol | English | Metric |
| \( h \) = height | \( in \) | \( mm \) |
| \( x \) = angle | \( deg \) | \( rad \) |
| \( l \) = length | \( in \) | \( mm \) |
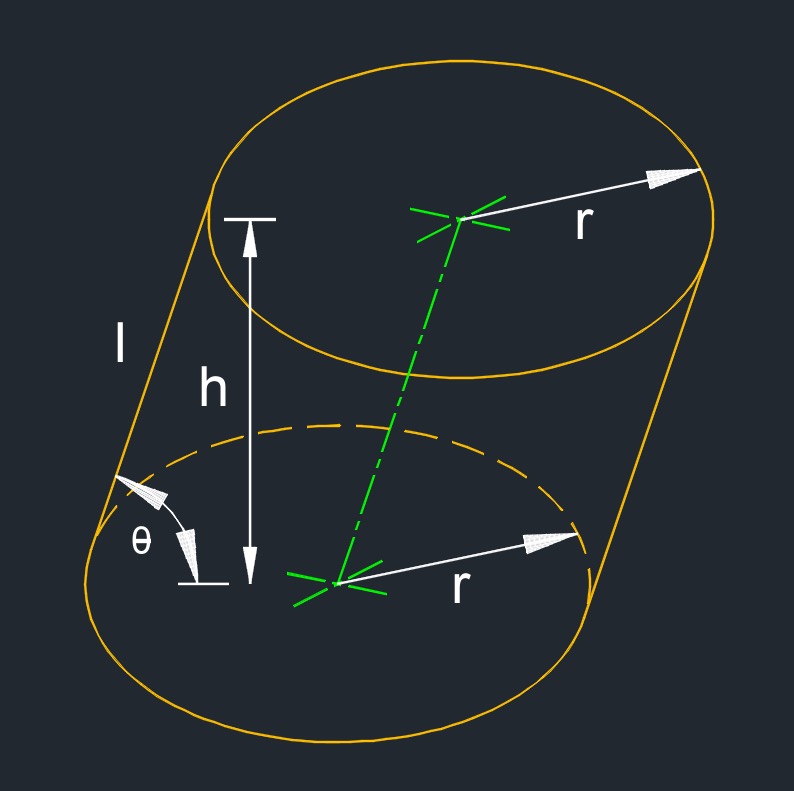
- Oblique cylinder (a three-dimensional figure) has both bases not alligned above each other and the center not at 90° to the other base center.
- 2 bases
Lateral surface area of a Oblique Cylinder formula |
||
| \( A_l \;=\; 2 \cdot \pi \cdot r \cdot l \) | ||
| Symbol | English | Metric |
| \( A_l \) = lateral surface area (side) | \( in^2 \) | \( mm^2 \) |
| \(\ l \) = length | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | \(3.141 592 653 ...\) |
| \( r \) = radius | \( in \) | \( mm \) |
Surface area of a Oblique Cylinder formula |
||
| \( A_s \;=\; l + 2 \cdot \pi \cdot r^2 \) | ||
| Symbol | English | Metric |
| \( A_s \) = surface area (bottom, top, side) | \( in^2 \) | \( mm^2 \) |
| \( l \) = length | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | \(3.141 592 653 ...\) |
| \( r \) = radius | \( in \) | \( mm \) |
Volume of a Oblique cylinder formula |
||
| \( V \;=\; \pi \cdot r^2 \cdot h \) | ||
| Symbol | English | Metric |
| \( V \) = volume | \( in^3 \) | \( mm^3 \) |
| \( h \) = height | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | \(3.141 592 653 ...\) |
| \( r \) = radius | \( in \) | \( mm \) |