Shear Stress

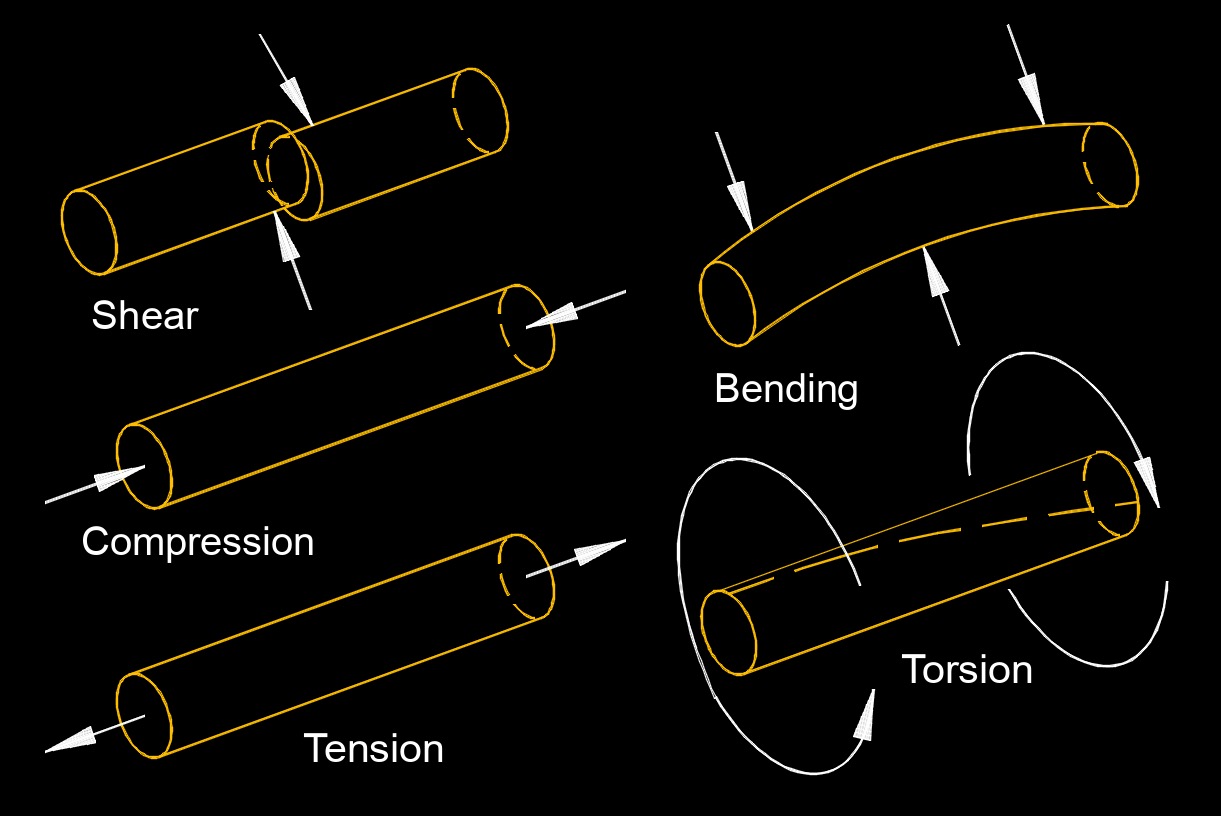Shear stress, abbreviated as \(\tau\) (Greek symbol tau), is a type of stress that occurs when a force is applied parallel to the surface of an object or material.  It is a measure of the internal resistance to the sliding or deformation of adjacent layers of a material when subjected to the applied force.  Shear stress is closely related to shear strain, which is the deformation or displacement of material layers relative to each other due to the applied force.  The relationship between shear stress and shear strain is described by the material's shear modulus or modulus of rigidity.

Different materials have different responses to shear stress.  Some materials, such as fluids, exhibit continuous deformation when subjected to shear stress and are called "shear-thinning" materials.  Others, like solids, have a more rigid response and resist deformation.

Shear stress plays a significant role in various fields, including physics, engineering, and geology.  It is crucial in the design and analysis of structures subjected to forces that cause shearing, such as beams, bridges, and mechanical components. In geology, shear stress is essential in understanding the behavior of rocks and faults during tectonic movements.

 

Shear stress formula
\( \tau \;=\; F \;/\; A_c \)     (Shear Stress) \( F \;=\; \tau \; A_c  \)  \( A_c \;=\; F \;/\; \tau \)
Symbol	English	Metric
\( \tau \)  (Greek symbol tau) = Shear Stress	\(lbf \;/\; in^2\)	\(Pa\)
\( F \) = Applied Force to the Material	\( lbf \)	\(N\)
\( A_c \) = Area Cross-section of Material Perpendicular to the Applied Force	\( in^2 \)	\( mm^2 \)