Magnetic Permeability

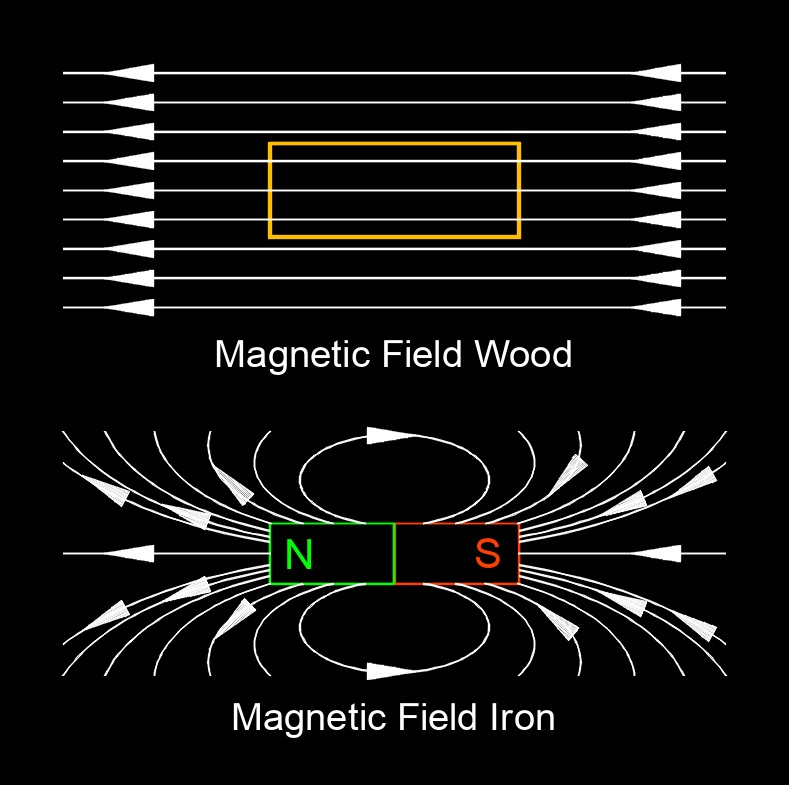Magnetic permeability, abbreviated as \(\mu\), also called permeability, is the ability of a material to respond to how much electromagnetic flux it can support to pass through itself within an applied electromagnetic field.

There are Three Main Categories of Magnetic Permeability

Materials can have different magnetic permeabilities depending on their composition and magnetic properties.

Diamagnetic Materials  -  These materials have a magnetic permeability slightly less than \(\mu_0\), and they exhibit a weak repulsion when placed in a magnetic field.  Diamagnetic materials create a magnetic field in the opposite direction to the applied field.  Examples of diamagnetic materials include bismuth, copper, and water.
Paramagnetic Materials  -  Paramagnetic materials have a magnetic permeability slightly greater than \(\mu_0\).  When placed in a magnetic field, paramagnetic materials become weakly magnetized in the same direction as the applied field.  Some common paramagnetic materials include aluminum, platinum, and oxygen.
Ferromagnetic Materials  -  Ferromagnetic materials have a significantly higher magnetic permeability than \(\mu_0\).  These materials exhibit strong magnetization when subjected to a magnetic field and can retain their magnetization after the field is removed.  Examples of ferromagnetic materials include iron, nickel, and cobalt.  Ferromagnetic materials are widely used in applications such as electromagnets, transformers, and magnetic storage devices.

It's important to note that the magnetic permeability of a material can vary with factors like temperature, applied magnetic field strength, and frequency of the magnetic field.  Magnetic permeability describes a material's ability to support or interact with magnetic fields.  It plays a crucial role in various fields of science and engineering, especially in applications involving magnets, electromagnetism, and magnetic materials.

 

Magnetic Permeability FORMULA
\( \mu \;=\; B\;/\;H\)     (Magnetic Permeability) \( B \;=\; \mu \; H  \) \( H \;=\; B\;/\;\mu\)
Symbol	English	Metric
\( \mu \)  (Greek symbol mu) = Magnetic Permeability	-	\(H\;/\;m\)
\( B \) = Total Magnetic Field Inside the Material (Magnetic Intensity)	-	\(T\)
\( H \) = Total Magnetic Field Outside the Material	-	\(T \)