Trigonometry
Mathematics, Glossary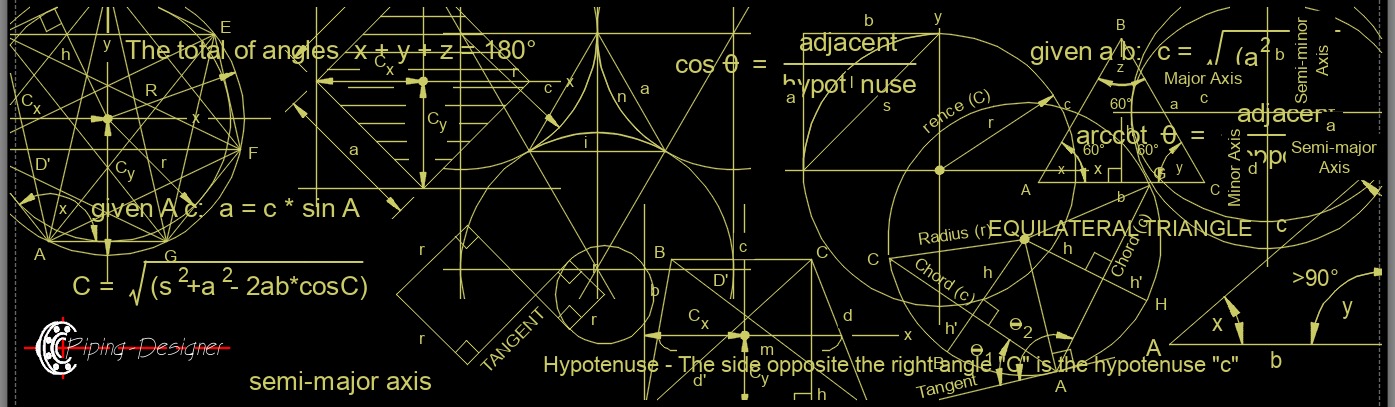 Trigonometry is a branch of mathematics that deals with the study of the relationships between the angles and sides of triangles. It is concerned with the measurement and calculation of angles and distances, and has many practical applications in fields such as physics, engineering, and astronomy. Trigonometry has many practical applications in fields such as engineering, physics, and navigation, where it is used to calculate distances, angles, and other geometric properties. It is also used in fields such as astronomy, where it is used to calculate the positions and movements of celestial objects.
Trigonometry is a branch of mathematics that deals with the study of the relationships between the angles and sides of triangles. It is concerned with the measurement and calculation of angles and distances, and has many practical applications in fields such as physics, engineering, and astronomy. Trigonometry has many practical applications in fields such as engineering, physics, and navigation, where it is used to calculate distances, angles, and other geometric properties. It is also used in fields such as astronomy, where it is used to calculate the positions and movements of celestial objects.
Trigonometry Glossary
A
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Arccosine - arccos, the inverse cosine.
- Arccotangent - arccot , the inverse cotangent.
- Arccosecant - arccsc, the inverse cosecant.
- Arcsecant - arcsec , the inverse secant.
- Arcsine - arcsin , the inverse sine.
- Arctangent - arctan , the inverse tangent.
B
C
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Cosecant - csc, the ratio of the hypotenuse over opposite side in a right triangle.
- Cosine - cos, the ratio of the adjacent side of the hypotenuse in a right triangle.
- Cotangent - cot, the ratio of the adjacent side to the oposite in a right triangle.
H
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Heron's Formula - A formula for the area of a triangle when the lengths of the three sides are known.
- Hyporenuse - The side of right triangle opposite the right angle, which is the longest side.
I
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Infinite - Having no boundary.
- Inverse Function - The function obtained by switching the \(\large{ x- }\) and \(\large{ y- }\) variables in a function. The inverse of function \(\large{ f }\) is \(\large{ f^{-1} }\) .
- Inverse Statement - Disaliow both the hypohesis and conclusion of the origional statement.
L
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Law of Cosines - An equation relating to cosine of an interior angle and the lengths of the sides of a triangle.
- Law of Sines - The ratio of the sine of any angle within any specific triangle and the length for the opposite side is a constant.
O
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Orthocenter - The point of concurrence of the altitudes of the triangle.
- Orthogonal - Perpendicular to a plane.
P
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Pythagorean Theorem - An equation relating the lengths of the sides of a right triangle. The sum of the squares of the legs of a right triangle is equal to the square of the hypotenuse.
- Proportional - In a constant ratio.
R
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Ratio - A constant proportion or two real numbers divided by each other.
S
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Secant - sec, the ratio of the hypotenuse to the side adjacent to a given angle in a right triangle.
- Sine - sin, the ratio of the length of the opposite side to the length of the hypotenuse.
T
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Tangent - The ratio of the opposite side to the adjacent side of a right triangle.
- Tangent Line - A line that touches the graph of the function at a single point.
- Tangent of a Circle - A line that contacts an arc or circle at only one point.
-
- sine = sin
- cosine = cos
- tangent = tan
- cotangent = cot
- cosecant = csc
- secant = sec
- arcsine = arcsin
- arccosine = arccos
- arctangent = arctan
- arccotangent = arccot
- arccosecant = arccsc
- arcsecant = arcsec
- Trig Functions - sine, cosine, tangent, cosecant, secant, and cotangent
X
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- X-axis - The horizontal axis on a coordinate system.
Y
- A - B - C - D - E - F - G - H - I - J - K - L - M - N - O - P - Q - R - S - T - U - V - W - X - Y - Z
- Y-axis - The vertical axis on a coordinate system.

