Heat Flow Rate
Heat Flow Rate Formula |
||
|
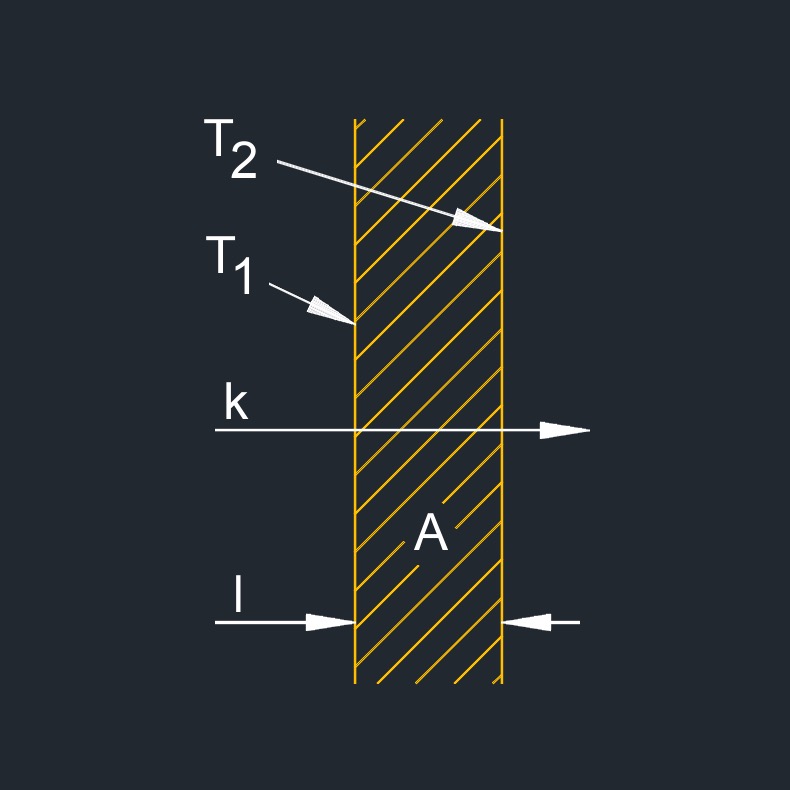
\( Q_f \;=\; - \; \left( k \cdot \dfrac{ A }{ l } \cdot \Delta T \right) \) (Heat Flow Rate) \( k \;=\; - \; \dfrac{ Q_f \cdot l }{ A \cdot \Delta T } \) \( A \;=\; - \; \dfrac{ Q_f \cdot l }{ k \cdot \Delta T } \) \( l \;=\; - \; \dfrac{ k \cdot A \cdot \Delta T }{ Q_f } \) \( \Delta T \;=\; - \; \dfrac{ Q_f \cdot l }{ k \cdot A } \) |
||
| Symbol | English | Metric |
| \( Q_f \) = Heat Flow Rate | \(Btu\;/\;hr\) | \(W\) |
| \( k \) or \( \lambda \) (Greek symbol lambda) = Thermal Conductivity | \(Btu-ft\;/\;hr-ft^2-F\) |
\(W\;/\;m-K\) |
| \( A \) = Emitting Body Area | \(ft^2\) | \(m^2\) |
| \( l \) = Material Length | \(ft\) | \(m\) |
| \( \Delta T \) = Temperature Differential (\(T_1 - T_2\)) | \(F\) | \(K\) |
| \( T_1 \) = Temperature of One Surface of the Wall | \(F\) | \(K\) |
| \( T_2 \) = Temperature of the Other Surface of the Wall | \(F\) | \(K\) |
 Heat flow rate, abbreviated as \(Q_f\), is a measure of the amount of heat energy transferred or conducted through a material or system per unit of time. It represents the rate at which thermal energy is moving from one region to another. Heat flow rate can be important in various applications and fields, including physics, engineering, and environmental science. It is used to quantify heat transfer in systems such as heat exchangers, buildings, electronic devices, and many other situations where understanding and controlling heat transfer is essential.
Heat flow rate, abbreviated as \(Q_f\), is a measure of the amount of heat energy transferred or conducted through a material or system per unit of time. It represents the rate at which thermal energy is moving from one region to another. Heat flow rate can be important in various applications and fields, including physics, engineering, and environmental science. It is used to quantify heat transfer in systems such as heat exchangers, buildings, electronic devices, and many other situations where understanding and controlling heat transfer is essential.

