Escape Velocity Formula |
||
|
\( v_e \;=\; \sqrt { \dfrac{ 2 \cdot G \cdot m }{ r } }\) (Escape Velocity) \( G \;=\; \dfrac{ v_e^2 \cdot r }{ 2 \cdot m }\) \( m \;=\; \dfrac{ v_e^2 \cdot r }{ 2 \cdot G }\) \( r \;=\; \dfrac{ 2 \cdot G \cdot m }{ v_e^2 }\) |
||
| Symbol | English | Metric |
| \( v_e \) = Escape Velocity | \(ft\;/\;sec\) | \(m\;/\;s\) |
| \( m \) = Mass of the Planet or Moon | \( lbm \) | \( kg \) |
| \( r \) = Radius from the Center of Mass (Planet or Moon) to Start Point | \( ft \) | \( m \) |
| \( G \) = Universal Gravitational Constant | \(lbf-ft^2\;/\;lbm^2\) | \(N - m^2\;/\;kg^2\) |
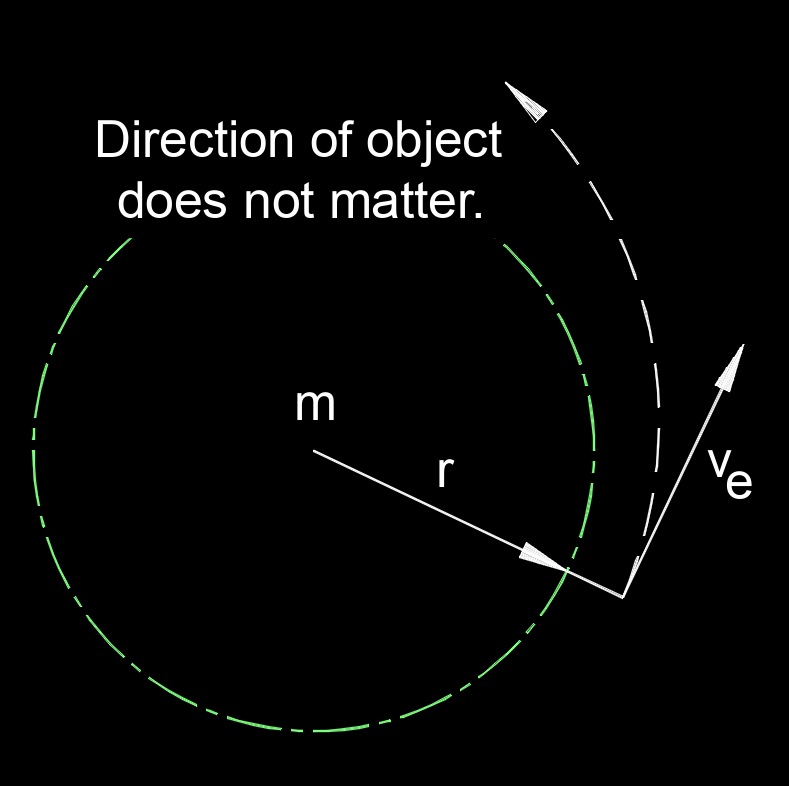Escape velocity, abbreviated as \(v_e\), is the minimum velocity an object must reach to break free from the gravitational pull of a celestial body, such as a planet or moon, and move into space without further propulsion. You can also say it is the speed required for an object to escape the gravitational influence of a massive body and move into a stable orbit or into open space.
The concept of escape velocity arises from Newton's law of universal gravitationn, which states that every object with mass exerts a gravitational force on other objects with mass. To escape the gravitational pull of a celestial body, an object must have enough kinetic energy to overcome this gravitational force.
Key Points about Escape Velocity
- It is independent of the mass of the escaping object. Whether it's a small spacecraft or a massive rocket, they all need to reach the same escape velocity to leave a celestial body.
- Escape velocity depends on the mass and radius of the celestial body. Larger and more massive bodies require higher escape velocities.
- Achieving escape velocity does not mean that an object will leave the celestial body's gravitational influence and travel indefinitely through space. Additional propulsion may be required to navigate in space, enter orbit around another body, or change its trajectory.
- Escape velocity is a critical factor in space exploration and rocketry. Spacecraft must be launched with sufficient speed to overcome Earth's gravitational pull and enter space, and similar considerations apply when leaving the gravitational influence of other celestial bodies.
For Earth, the approximate escape velocity at the planet's surface is about 11.2 kilometers per second (km/s) or approximately 25,020 miles per hour (40,270 kilometers per hour). This value decreases with increasing altitude above the Earth's surface since the gravitational force weakens with distance from the center of the planet.
