Darcy Friction Factor - Laminar Flow Formula |
||
|
\( f_l \;=\; \dfrac{ 64 }{ Re }\) (Darcy Friction Factor - Laminar Flow) \( Re \;=\; \dfrac{ 64 }{ f_l }\) |
||
| Symbol | English | Metric |
| \( f_l \) = Darcy friction factor laminar flow | \( dimensionless \) | \( dimensionless \) |
| \( Re \) = Reynolds number | \( dimensionless \) | \( dimensionless \) |
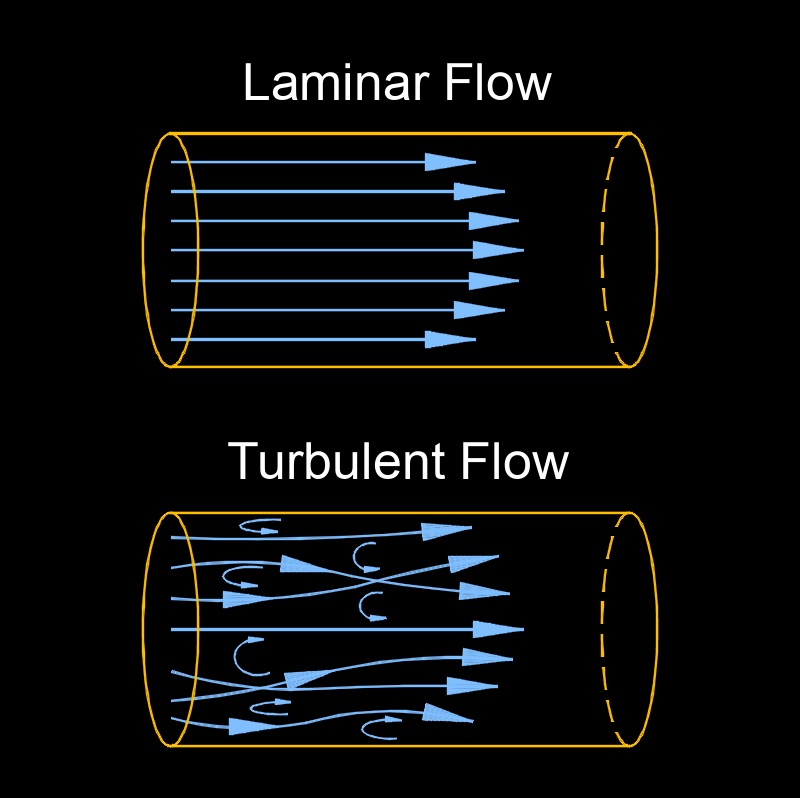Darcy friction factor formulas, a dimensionless number, is used in fluid mechanics to describe the friction losses in pipe flow due to the shear stress between the fluid and the pipe walls. For laminar flow, which occurs when the flow is smooth and orderly (typically at low Reynolds numbers, Re < 2000, the Darcy friction factor has a specific, exact relationship with the Reynolds number.
Key Points abour Darcy Friction Factor - Laminar Flow
Dependence on Reynolds Number - The factor \( f = \frac{64}{Re }shows that the friction factor is inversely proportional to the Reynolds number. As the flow becomes slower or more viscous (lower Re), the friction factor increases.
Laminar Flow Only - This formula is valid only for laminar flow conditions. For turbulent flow (Re > 4000), the friction factor depends on both the Reynolds number and the pipe's relative roughness, and it’s typically determined using empirical correlations or the Moody chart.
Physical Meaning - The Darcy Friction Factor quantifies the energy loss due to friction per unit length of pipe, which is critical for calculating pressure drops in systems (via the Darcy-Weisbach equation).
