Two Span Continuous Beam - Unequal Spans, Uniformly Distributed Load
- See Article - Beam Design Formulas
Span Continuous Beam - Unequal Spans, Uniformly Distributed Load formulas |
||
|
\( R_1 \;=\; V_1 \;=\; \dfrac{ M_1 }{ a } + \dfrac{ w\cdot a }{ 2 } \) \( R_2 \;=\; w\cdot a + w\cdot b - R_1 - R_3 \) \( R_3 \;=\; V_4 \;=\; \dfrac{ M_1 }{ b } + \dfrac{ w\cdot a }{ 2 } \) \(V_2 \;=\; w\cdot a - R_1 \) \( V_3 \;=\; w\cdot b - R_3 \) \( M_1 \;=\; \dfrac{ w\cdot b^3 + w\cdot a^3 }{ 8 \cdot ( a+b ) } \) \( M_1 \;=\; \dfrac{ w\cdot b^3 + w\cdot a^3 }{ 8 \cdot ( a+ b) } \) \( M_{x_2} \; ( x_2 = \frac{R_3}{w} ) \;=\; R_3 \cdot x_2 - \dfrac{ w\cdot x_2^2 }{ 2 } \) |
||
| Symbol | English | Metric |
| \( \Delta \) = deflection or deformation | \(in\) | \(mm\) |
| \( x \) = horizontal distance from reaction to point on beam | \(in\) | \(mm\) |
| \( w \) = load per unit length | \(lbf\;/\;in\) | \(N\;/\;m\) |
| \( M \) = maximum bending moment | \(lbf-in\) | \(N-mm\) |
| \( V \) = maximum shear force | \(lbf\) | \(N\) |
| \( R \) = reaction load at bearing point | \(lbf\) | \(N\) |
| \( a, b \) = span length under consideration | \(in\) | \(mm\) |
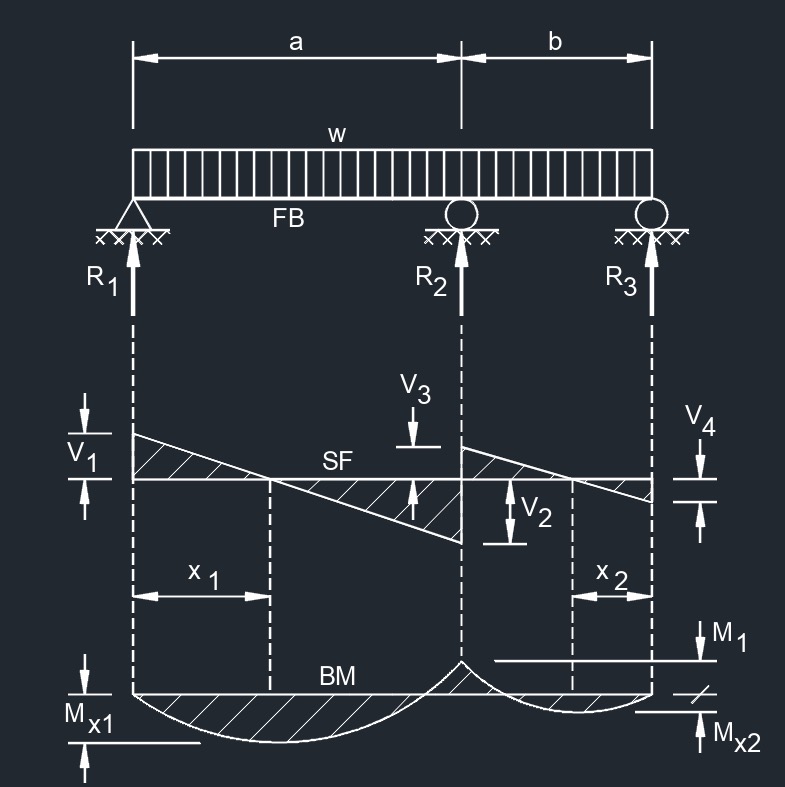
Diagram Symbols
Bending moment diagram (BMD) - Used to determine the bending moment at a given point of a structural element. The diagram can help determine the type, size, and material of a member in a structure so that a given set of loads can be supported without structural failure.
Free body diagram (FBD) - Used to visualize the applied forces, moments, and resulting reactions on a structure in a given condition.
Shear force diagram (SFD) - Used to determine the shear force at a given point of a structural element. The diagram can help determine the type, size, and material of a member in a structure so that a given set of loads can be supported without structural failure.
Uniformly distributed load (UDL) - A load that is distributed evenly across the entire length of the support area.

