Thin Wall Circle
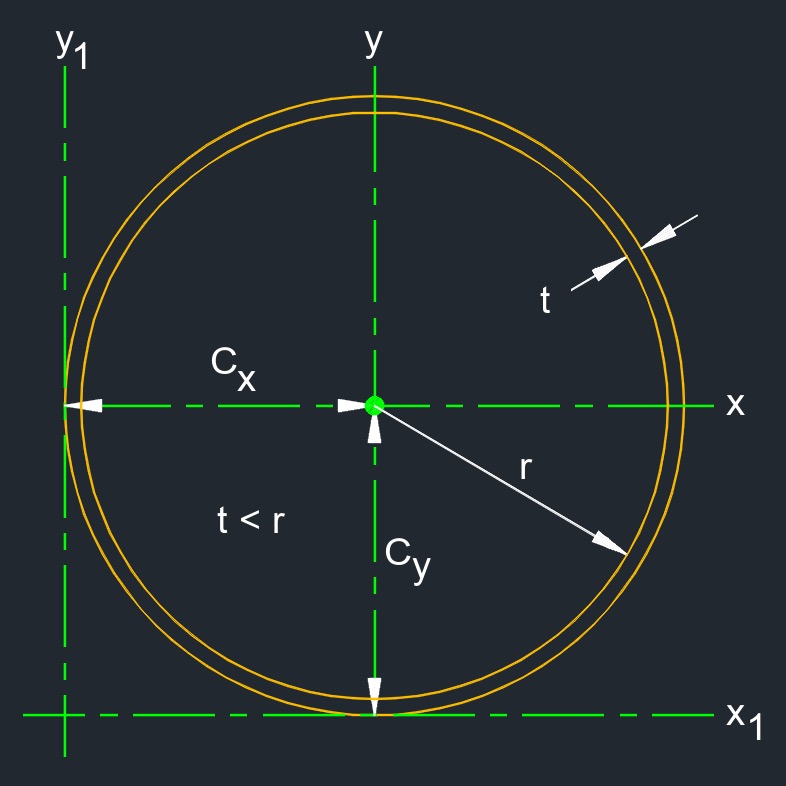Two circles each having all points on each circle at a fixed equal distance from a center point.- Center of a circle having all points on the line circumference are at equal distance from the center point.
- A thin wall circle is a structural shape used in construction.
- See Article Link - Geometric Properties of Structural Shapes
- Tags: Structural Steel Circle
Thin Wall Circle Index
- Area of a Thin Walled Circle
- Perimeter of a Thin Walled Circle
- Radius of a Thin Walled Circle
- Distance from Centroid of a Thin Walled Circle
- Elastic Section Modulus of a Thin Walled Circle
- Plastic Section Modulus of a Thin Walled Circle
- Polar Moment of Inertia of a Thin Walled Circle
- Radius of Gyration of a Thin Walled Circle
- Second Moment of Area of a Thin Walled Circle
- Torsional Constant of a Thin Walled Circle
area of a Thin Walled Circle formula |
||
| \( A \;=\; 2\; \pi \;r\; t \) | ||
| Symbol | English | Metric |
| \( A \) = area | \( in^2 \) | \( mm^2 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Perimeter of a Thin Walled Circle formula |
||
|
\( P \;=\; 2\; \pi \;r \) (outside) \( P \;=\; 2\; \pi \; ( r - t ) \) (inside) |
||
| Symbol | English | Metric |
| \( P \) = perimeter | \( in \) | \( mm \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Radius of a Thin Walled Circle formula |
||
|
\( r \;=\; \sqrt{ 2 \; A \;/\; \pi } \) (Radius of a Thin Walled Circle) \( A \;=\; r^2 \; \pi \;/\; 2 \) |
||
| Symbol | English | Metric |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( A \) = area | \( in^2 \) | \( mm^2 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
Distance from Centroid of a Thin Walled Circle formulas |
||
|
\( C_x \;=\; r \) \( C_y \;=\; r \) |
||
| Symbol | English | Metric |
| \( C_x, C_y \) = distance from centroid | \( in \) | \( mm \) |
| \( r \) = inside radius | \( in \) | \( mm \) |
Elastic Section Modulus of a Thin Walled Circle formula |
||
|
\( S \;=\; 2 \; \pi \; r \; t \;/\; 3 \) (Elastic Section Modulus of a Thin Walled Circle) \( r \;=\; S \; 3 \;/\; 2 \; \pi \; t \) \( t \;=\; S \; 3 \;/\; 2 \; \pi \; r \) |
||
| Symbol | English | Metric |
| \( S \) = elastic section modulus | \( in^3 \) | \(mm^3\) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Plastic Section Modulus of a Thin Walled Circle formula |
||
|
\( Z \;=\; \pi \; r^2 \; t \) (Plastic Section Modulus of a Thin Walled Circle) \( r \;=\; \sqrt{ Z \;/\; \pi \; t } \) \( t \;=\; Z \;/\; \pi \; r^2 \) |
||
| Symbol | English | Metric |
| \( Z \) = plastic section modulus | \( in^3 \) | \(mm^3 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Polar Moment of Inertia of a Thin Walled Circle formulas |
||
|
\( J_{z} \;=\; 2\; \pi \;r^3 \;t \) \( J_{z1} \;=\; 6\; \pi \;r^3 \;t \) |
||
| Symbol | English | Metric |
| \( J \) = torsional constant | \( in^4 \) | \(mm^4 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Radius of Gyration of a Thin Walled Circle formulas |
||
|
\( k_{x} \;=\; ( \sqrt {2} \;/\; 2 ) \; r \) \( k_{y} \;=\; ( \sqrt {2} \;/\; 2 ) \; r \) \( k_{z} \;=\; r \) \( k_{x1} \;=\; ( \sqrt {6} \;/\; 2 ) \; r \) \( k_{y1} \;=\; ( \sqrt {6} \;/\; 2 ) \; r \) \( k_{z1} \;=\; \sqrt {3} \; r \) |
||
| Symbol | English | Metric |
| \( k \) = radius of gyration | \( in \) | \( mm \) |
| \( r \) = inside radius | \( in \) | \( mm \) |
Second Moment of Area of a Thin Walled Circle formulas |
||
|
\( I_{x} \;=\; \pi \;r^3 \;t \) \( I_{x1} \;=\; 3\; \pi \;r^3 \;t \) \( I_{y1} \;=\; 3\; \pi \;r^3 \;t \) |
||
| Symbol | English | Metric |
| \( I \) = moment of inertia | \( in^4 \) | \(mm^4 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Torsional Constant of a Thin Walled Circle formula |
||
|
\( J \;=\; 2\; \pi \;r^3 \; t \) (Torsional Constant of a Thin Walled Circle) \( r \;=\; ( J \;/\; 2 \; \pi \; t )^{ \frac{1}{3} } \) \( t \;=\; J \;/\; 2 \; \pi \; r^3 \) |
||
| Symbol | English | Metric |
| \( J \) = torsional constant | \( in^4 \) | \(mm^4 \) |
| \( \pi \) = Pi | \(3.141 592 653 ...\) | |
| \( r \) = inside radius | \( in \) | \( mm \) |
| \( t \) = thickness | \( in \) | \( mm \) |
Tags: Structural Steel Circle