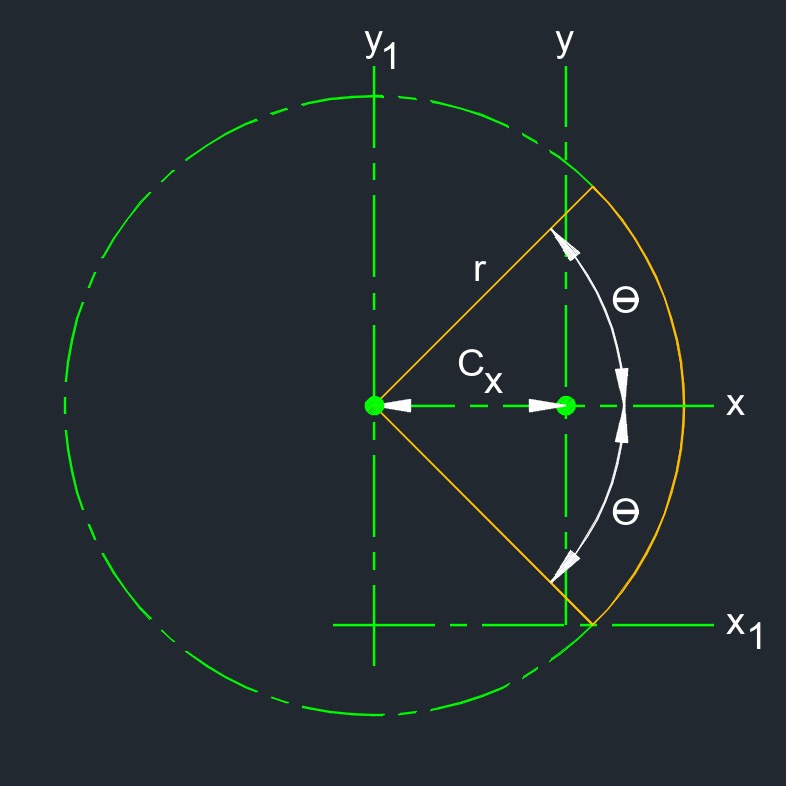
Sector of a Circle

Arc Length of a Sector formula
\( L \;=\; \Delta \cdot \dfrac{ \pi }{ 180 } \cdot r \)
Symbol	English	Metric
\( L \) = arc length	\( in \)	\(mm \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( \pi \) = Pi	\(3.141 592 653 ...\)	\(3.141 592 653 ...\)
\( r \) = radius	\( in \)	\( mm \)

Sector is a fraction of the area of a circle with a radius on each side and an arc.
Angle (\(\Delta\)) - Two rays sharing a common point.
Center (cp) - Having all points on the line circumference are at equal distance from the center point.
Chord (c) - Also called long chord (LC), is between any two points on a circular curve.
Circumference (C) - The outside of a circle or a complete circular arc.
Height (h) - Length of radius from radius center to midpoint of chord.

area of a Sector formula
\( A \;=\; \dfrac{ \Delta \cdot r^2 }{ 2 }\) \( A \;=\; \dfrac{ \Delta }{ 360 } \cdot \pi \cdot r^2 \) \( A \;=\; \dfrac{ \Delta \cdot \pi }{ 360 } \cdot r^2 \)
Symbol	English	Metric
\( A \) = area of sector	\( in^2 \)	\(mm^2 \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( \pi \) = Pi	\(3.141 592 653 ...\)	\(3.141 592 653 ...\)
\( r \) = radius	\( in \)	\( mm \)

Height (h') - Length of radius from midpoint of chord to point on circular curve.
Length (L) - Total length of any circular curve measured along the arc.
Radius (r) - Half the diameter of a circle. A line segment between the center point and a point on a circle or sphere.
Radius Point (rp) - Radius center point of circular curve.
Segment is an interior part of a circle bound by a chord and an arc.
Tangent (T) - A line that touches a curve at just one point such that it is perpendicular to a radius line of the curve.
See Article Links - Geometric Properties of Structural Shapes

Distance from Centroid of a Sector formulas
\( C_x \;=\; 2 \cdot r \cdot \dfrac{ sin(\Delta) }{ 3 \cdot \Delta } \) \( C_y \;=\; 0 \)
Symbol	English	Metric
\( C \) = distance from centroid	\( in \)	\( mm \)
\( A \) = area of sector	\( in \)	\( mm \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( r \) = radius	\( in \)	\( mm \)

Elastic Section Modulus of a Sector formula
\( S \;=\; \dfrac{ I_x }{ sin(\Delta) \cdot r }\)
Symbol	English	Metric
\( S \) = elastic section modulus	\( in^3 \)	\( mm^3 \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( I \) = moment of inertia	\( in^4 \)	\( mm^4 \)
\( r \) = radius	\( in \)	\( mm \)

Perimeter of a Sector formula
\( P \;=\; 2 \cdot r + 2 \cdot r \cdot \Delta \)
Symbol	English	Metric
\( P \) = perimeter	\( in \)	\( mm \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( r \) = radius	\( in \)	\( mm \)

Polar Moment of Inertia of a Sector formulas
\( J_{z} \;=\; \dfrac{ r^4 }{ 18 } \cdot \dfrac{ 9 \cdot \Delta^2 - 8 \cdot sin^2(\Delta) }{ \Delta }\) \( J_{z1} \;=\; \dfrac{ r^4 \cdot \Delta }{ 2 } \)
Symbol	English	Metric
\( J \) = torsional constant	\( in^4 \)	\( mm^4 \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( r \) = radius	\( in \)	\( mm \)

Radius of Gyration of a Sector formulas
\( k_{x} \;=\; \dfrac{1}{4} \cdot \sqrt{ 2 \cdot r^2 \cdot \dfrac{ 2\cdot \Delta - sin(2 \cdot \Delta ) }{ \Delta } } \) \( k_{y} \;=\; \dfrac{1}{12} \cdot \sqrt{ 2 \cdot r^2 \cdot \dfrac{ 180^2 + 9\cdot \Delta \cdot sin(2\cdot \Delta) - 32 + 32 \cdot cos^2(\Delta) }{ \Delta^2 } } \) \( k_{z} \;=\; \dfrac{1}{6} \cdot \sqrt{ 2 \cdot r^2 \cdot \dfrac{ 9 \cdot \Delta^2 - 8 \cdot sin^2(2\cdot \Delta ) }{ \Delta^2 } } \) \( k_{x1} \;=\; \dfrac{1}{4} \cdot \sqrt{ 2 \cdot r^2 \cdot \dfrac{ 2\cdot \Delta - sin(2 \cdot \Delta) }{ \Delta } } \) \( k_{y1} \;=\; \dfrac{1}{4} \cdot \sqrt{ 2 \cdot r^2 \cdot \dfrac{ 2\cdot \Delta + sin(2 \cdot \Delta ) }{ \Delta } } \) \( k_{x1} \;=\; \dfrac{ r }{ \sqrt{2} } \)
Symbol	English	Metric
\( k \) = radius of gyration	\( in \)	\( mm \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( r \) = radius	\( in \)	\( mm \)

Second Moment of Area of a Sector formulas
\( I_{x} \;=\; \dfrac{ r^4 }{ 4} \cdot \left( \Delta - \dfrac{1}{2} \cdot sin( 2 \cdot \Delta ) \right) \) \( I_{y} \;=\; \dfrac{ r^4 }{ 4} \cdot \left( \Delta + \dfrac{1}{2} \cdot sin( 2 \cdot \Delta ) \right) - \left( \dfrac{ 4 \cdot r^4 }{ 9 \cdot \Delta } \cdot sin^2 ( \Delta ) \right) \) \( I_{x1} \;=\; I_x + r^4 \cdot \Delta \cdot sin^2 (\Delta) \) \( I_{y1} \;=\; \dfrac{ r^4 }{ 4} \cdot \left( \Delta + \dfrac{1}{2} \cdot sin( 2 \cdot \Delta ) \right) \)
Symbol	English	Metric
\( I \) = moment of inertia	\( in^4 \)	\( mm^4 \)
\( \Delta \) = angle	\( deg \)	\(rad \)
\( r \) = radius	\( in \)	\( mm \)