Linear Spring Shear Stress

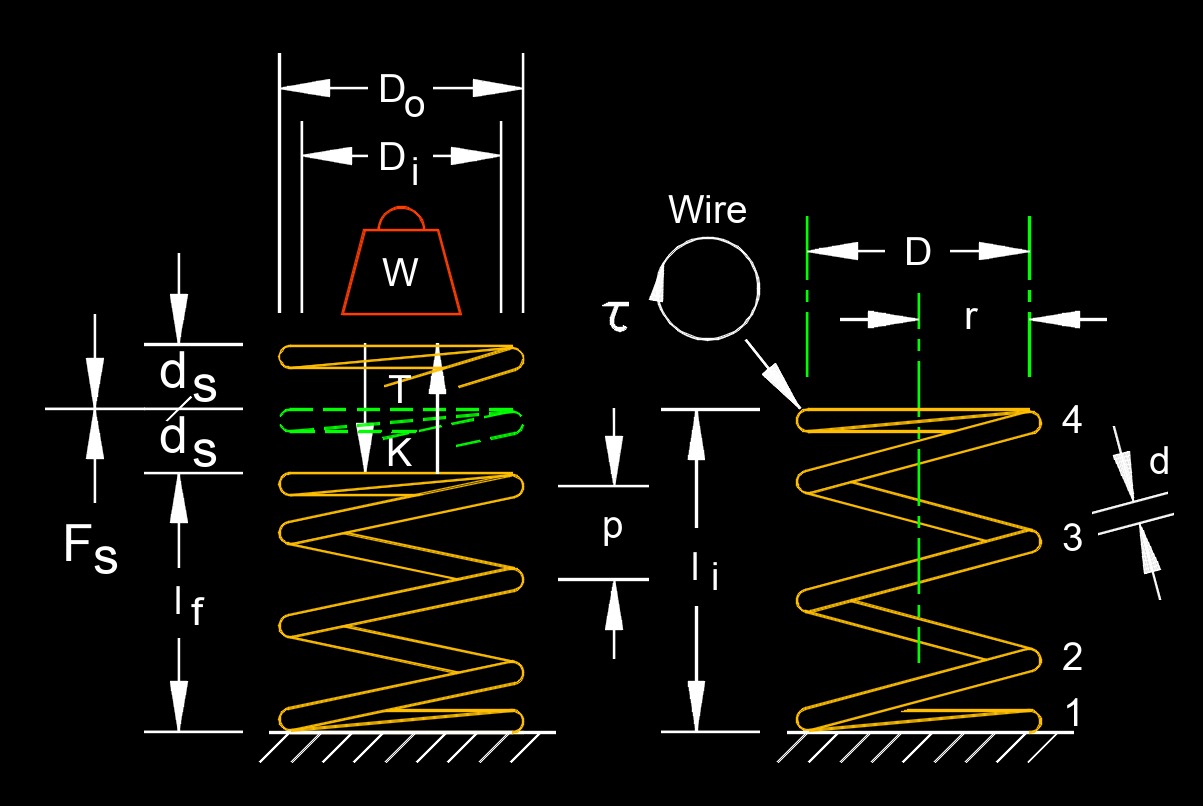Linear spring shear stress is the stress experienced by a material or a structure due to the application of a shear force, specifically in the context of linear springs.

Linear springs are mechanical components designed to store and release energy when subjected to a force, typically in the form of tension or compression.  When a linear spring is subjected to a shear force, the material experiences shear stress, which is a measure of the internal resistance of the material to the applied force that tries to deform or shear it.

Understanding the shear stress in linear springs is needed for designing and analyzing mechanical systems and ensuring that the springs can withstand the applied loads without experiencing failure or excessive deformation.  It's worth noting that the linear spring shear stress is directly proportional to the applied force and inversely proportional to the area cross-sectional of the spring.

   

 

Helical Linear Spring Shear Stress formula
\( \tau = k_s \; ( 8 \; f_a \; D \;/\; \pi \; d^3 ) \)
Symbol	English	Metric
\( \tau \) (Greek symbol tau) = shear stress	\(lbf\;/\;in^2\)	\(Pa\)
\( k_s \) = spring constant	\(lbf\)	\(N\)
\( f_a \) = applied force	\(lbf\)	\(N\)
\( D \) = mean spring coil diameter	\(in\)	\(mm\)
\( \pi \) = Pi	\(3.141 592 653 ...\)
\( d \) = wire diameter	\(in\)	\(mm\)