Angular Momentum
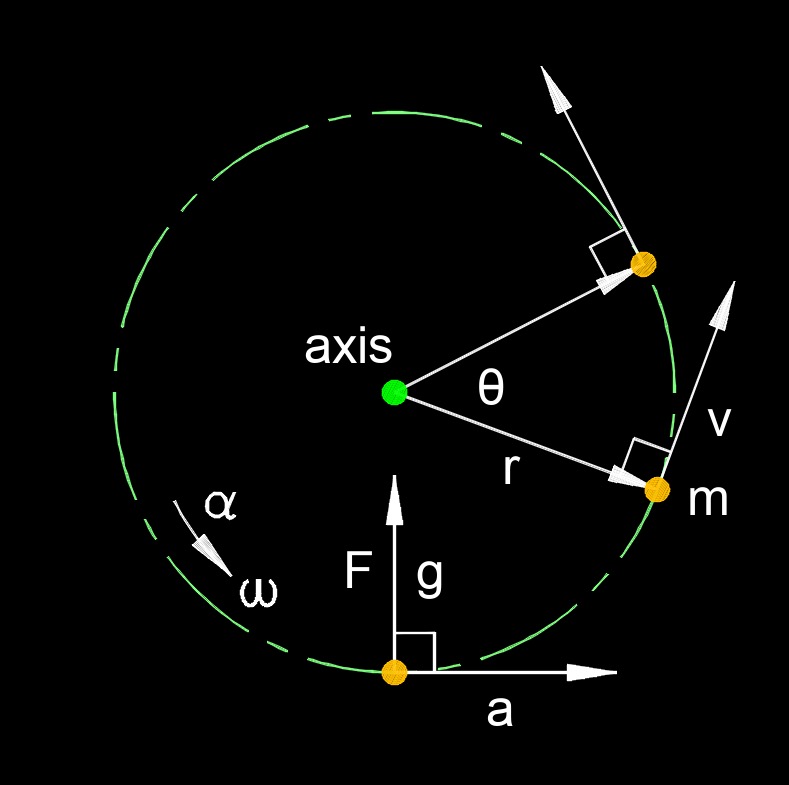Angular momentum, abbreviated as L, also called rotational momentum or moment of momentum, is how much an object is rotating around a fixed point. The angular momentun of a body is equal to the mass of the body multiplied by the cross product of the position vector of the particle with its vertical velocity.
It is a vector quantity that represents the amount of rotational motion an object possesses. Angular momentum is conserved in an isolated system when no external torques are acting on it. This is known as the conservation of angular momentum. According to this principle, the total angular momentum of a system remains constant unless influenced by external torques.
The conservation of angular momentum has important implications in various physical phenomena. For example, it explains why a spinning ice skater can increase or decrease their rotation speed by changing their body position. When the skater extends their arms, their moment of inertia increases, resulting in a decrease in angular velocity to conserve angular momentum. Conversely, when the skater pulls their arms inward, their moment of inertia decreases, leading to an increase in angular velocity.
Angular momentum is also relevant in celestial mechanics, where it explains phenomena such as the conservation of rotational motion in rotating planets and the stability of spinning objects in space.
Angular Momentum Moment of Inertia Formula |
||
|
\( L \;=\; I \cdot \omega \) (Angular Momentum) \( I \;=\; \dfrac{ L }{ \omega }\) \( \omega \;=\; \dfrac{ L }{ I }\) |
||
| Symbol | English | Metric |
| \( L \) = Angular Momentum (Rotational Momentum) | \(lbm - ft^2 \;/\; sec\) | \(kg - m^2 \;/\; s\) |
| \( I \) = Moment of Inertia | \(in^4\) | \(m^4\) |
| \( \omega \) (Greek symbol omega) = Angular Velocity | \(deg \;/\; sec\) | \(rad \;/\; s\) |
Angular Momentum mass Formula |
||
|
\( L \;=\; m \cdot v \cdot r \) (Angular Momentum) \( m \;=\; \dfrac{ L }{ v \cdot r }\) \( v \;=\; \dfrac{ L }{ m \cdot r } \) \( r \;=\; \dfrac{ L }{ m \cdot v } \) |
||
| Symbol | English | Metric |
| \( L \) = Angular Momentum (Rotational Momentum) | \(lbm - ft^2 \;/\; sec\) | \(kg - m^2 \;/\; s\) |
| \( m \) = Mass | \(lbm\) | \(kg\) |
| \( v \) = Velocity | \(ft \;/\; sec\) | \(m \;/\; s\) |
| \( r \) = Radius | \(ft\) | \(m\) |
Angular Momentum Radius Formula |
||
|
\( L \;=\; r \cdot p \) (Angular Momentum) \( r \;=\; \dfrac{ L }{ p }\) \( p \;=\; \dfrac{ L }{ r }\) |
||
| Symbol | English | Metric |
| \( L \) = Angular Momentum (Rotational Momentum) | \(lbm - ft^2 \;/\; sec\) | \(kg - m^2 \;/\; s\) |
| \( r \) = Radius | \(ft\) | \(m\) |
| \( p \) = Momentum | \(lbm - ft \;/\; sec\) | \(kg - m \;/\; s\) |
